A*寻路算法（lua）
Published in：2024-03-26 | category： 算法 reading：

参考资料：

https://www.cnblogs.com/f-society/p/6818665.html

https://www.jianshu.com/p/a8950fa19b72

举例：

现有如下寻路网格，求从A点（5, 1）到B点（5, 9）的A*路径

灰色代表不可到达的格子，每次行动可以从格子的周围8个方向行进，代价如下图

不可穿越斜墙，例如下图中，从P点出发的8个方向里，标记为”NO”的点是不可直接到达的

算法代码

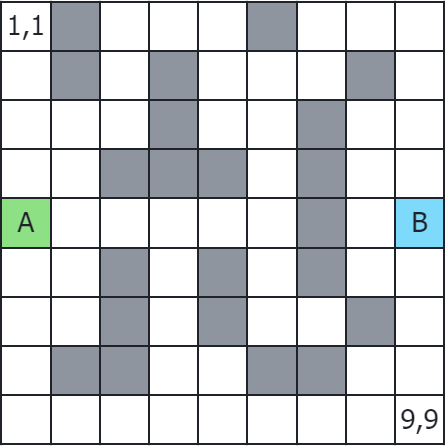
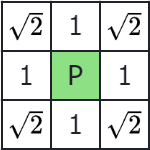
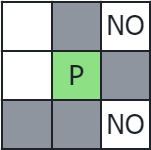
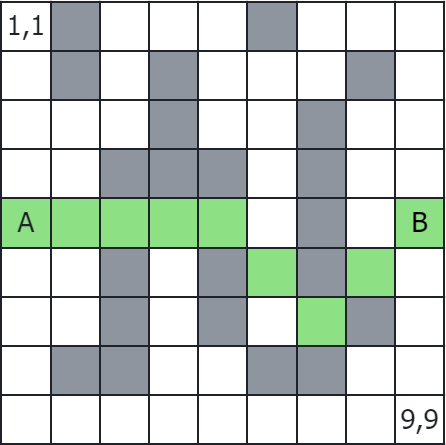
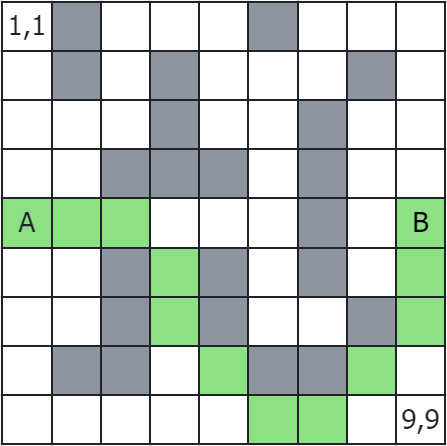
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
--定义方法，从列表中移除指定元素
local removeFromList = function(nodeList, element)
    for i = 1, #nodeList do
        if nodeList[i] == element then
            table.remove(nodeList, i)
            break
        end
    end
end

--定义方法：在列表中查找指定nodeIndex的元素
local findNodeByIndex = function(nodeList, nodeIndex)
    for i = 1, #nodeList do
        if nodeList[i].index == nodeIndex then
            return nodeList[i]
        end
    end
    return nil
end

--定义方法：判断列表是否存在指定元素
local contains = function(nodeList, nodeIndex)
    return findNodeByIndex(nodeList, nodeIndex) ~= nil
end

--定义方法：查找list中F值最小的结点
local findMinFNode = function(nodeList)
    local minF = math.maxinteger
    local minFNode = nil
    for i = 1, #nodeList do
        local n = nodeList[i]
        local F = n.G + n.H
        if F < minF then
            minF = F
            minFNode = n
        end
    end
    return minFNode
end

--构造一个node数据结构
local node = function(index, preNodeIndex, G, H)
    if index == nil then index = 0 end
    if preNodeIndex == nil then preNodeIndex = 0 end
    if G == nil then G = 0 end
    if H == nil then H = 0 end
    local n =
    {
        index = index,
        preNodeIndex = preNodeIndex,
        G = G,
        H = H,
    }
    return n
end

--构建9x9地图（用一维数组描述）
local map = 
{
    1,0,1,1,1,0,1,1,1,
    1,0,1,0,1,1,1,0,1,
    1,1,1,0,1,1,0,1,1,
    1,1,0,0,0,0,0,1,1,
    1,1,1,1,1,1,0,1,1,
    1,1,0,1,0,1,0,1,1,
    1,1,0,1,0,1,1,0,1,
    1,0,0,1,1,0,0,1,1,
    1,1,1,1,1,1,1,1,1,
}

local mapH = 9				--地图的横宽（Horizontal）
local mapV = #map / mapH	--地图的纵高（Vertical）
local mapDataLength = #map

local openList = {}		--搜索域（开启列表，存放待搜索的结点）
local closeList = {}	--已搜索域（关闭列表，存放已搜索的结点）

local startNodeIndex = 37		--指定一个起点
local endNodeIndex = 45			--指定一个终点
local openObliqueCheck = true	--是否开启斜墙判断，比如结点的上结点和右节点是墙，那右上结点就算不是墙，也不可通行
local minFNode = nil			--临时变量

--定义方法：计算一个结点的H值（这里使用曼哈顿距离，H = 当前结点到结束点的水平距离 + 当前结点到结束点的垂直距离）
local calculateH = function(nodeIndex)
    local projectedXEnd = (endNodeIndex - 1) % mapH + 1		--取endNodeIndex在首行的投影，例如  44->8  45->9  46->1
    local projectedXNode = (nodeIndex - 1) % mapH + 1		--取nodeIndex在首行的投影
    local projectedYEnd = endNodeIndex - (endNodeIndex - 1) % mapH	--取endNodeIndex在所在行首列的投影，例如  44->37  45->37  46->46
    local projectedYNode = nodeIndex - (nodeIndex - 1) % mapH		--取nodeIndex在所在行首列的投影
    local xDis = math.abs(projectedXEnd - projectedXNode)				--计算两点的水平距离
    local yDis = math.abs(projectedYEnd - projectedYNode) / mapH		--计算两点垂直距离
    return xDis + yDis
end

--定义方法：判断一个点是否是可通行的
local isReachable = function(nodeIndex)
    return nodeIndex > 0 and nodeIndex <= mapDataLength and map[nodeIndex] > 0
end

--初始化数据
table.insert(openList, node(startNodeIndex, -1, 0, calculateH(startNodeIndex)))	--首先把起点放进搜索域

repeat
    --在openList中查找F（F = G + H）值最低的结点
    minFNode = findMinFNode(openList)
    --遍历这个结点周围一圈8个结点，筛选出可行进的+未在开启列表+未在关闭列表的结点，放入开启列表
    --使用便于理解的命名方式
    local upLeft = minFNode.index - mapH - 1	--左上点（值<1代表已越界）
    if upLeft % mapH == 0 then upLeft = -1 end	--minFNode位于最左边时，左上点不存在
    local up = minFNode.index - mapH			--上点（值<1代表已越界）
    local upRight = minFNode.index - mapH + 1	--右上点（值<1代表已越界）
    if upRight % mapH == 1 then upRight = -1 end--minFNode位于最右边时，右上点不存在
    local left = minFNode.index - 1				--左点（值<1代表已越界）
    if left % mapH == 0 then left = -1 end		--minFNode位于最左边时，左点不存在
    local right = minFNode.index + 1			--右点（值>mapDataLength代表已越界）
    if right % mapH == 1 then right = -1 end	--minFNode位于最右边时，右点不存在
    local bottomLeft = minFNode.index + mapH - 1--左下点（值>mapDataLength代表已越界）
    if bottomLeft % mapH == 0 then bottomLeft = -1 end	--minFNode位于最左边时，左下点不存在
    local bottom = minFNode.index + mapH		--下点（值>mapDataLength代表已越界）
    local bottomRight = minFNode.index + mapH + 1--右下点（值>mapDataLength代表已越界）
    if bottomRight % mapH == 1 then bottomRight = -1 end--minFNode位于最右边时，右下点不存在

    --放入列表
    local neighborList = {upLeft, up, upRight, left, right, bottomLeft, bottom, bottomRight}

    --斜墙判断
    local obliqueReachableList = {1,1,1,1,1,1,1,1}		--和neighborList一样按照从左至右，从上至下记录周围结点是否可达
    if openObliqueCheck then
        local isReachable_up = isReachable(up)
        local isReachable_left = isReachable(left)
        local isReachable_right = isReachable(right)
        local isReachable_bottom = isReachable(bottom)
        if (not isReachable_left) and (not isReachable_up) then obliqueReachableList[1] = 0 end		--左上角不可达
        if (not isReachable_up) and (not isReachable_right) then obliqueReachableList[3] = 0 end		--右上角不可达
        if (not isReachable_left) and (not isReachable_bottom) then obliqueReachableList[6] = 0 end	--左下角不可达
        if (not isReachable_bottom) and (not isReachable_right) then obliqueReachableList[8] = 0 end	--右下角不可达
    end

    for i = #neighborList, 1, -1 do
        --剔除越界的点，剔除不可通行的点，剔除已在开放列表的点，剔除已在关闭列表的点
        local n = neighborList[i]
        local reachable = isReachable(n)
        if (not reachable) or obliqueReachableList[i] == 0 or contains(openList, n) or contains(closeList, n) then
            table.remove(neighborList, i)
        else
            --计算好G和H值后放入openList中（上下左右步长按1计算，四个角按照1.414计算）
            local stepValue = 1
            if i == 1 or i == 3 or i == 6 or i == 8 then stepValue = 1.414 end
            local node = node(n, minFNode.index, minFNode.G + stepValue, calculateH(n))
            table.insert(openList, node)
        end
    end
    --把minFNode从openList转移到closeList中
    removeFromList(openList, minFNode)
    table.insert(closeList, minFNode)

until(minFNode.index == endNodeIndex or #openList == 0)	--当openList为空或者找到终点时，算法结束

--在closeList中取endNode进行回溯，直到找到startNode，再将这条回溯链路反转即为从startNode到endNode的A*寻路结果
local nodeIndex = endNodeIndex
local path = {}		--记录最短路径的结点
if contains(closeList, nodeIndex) then
    while true do
        node = findNodeByIndex(closeList, nodeIndex)
        table.insert(path, node)
        nodeIndex = node.preNodeIndex
        if nodeIndex == -1 then
            break
        end
    end
    --结果输出

    --定义方法：将一维数组的index转为更容易看的二维坐标
    local toVectorFormat = function(index)
        local x = index%mapH
        if x == 0 then x = mapH end
        return "("..math.ceil(index/mapH)..", "..x..")"
    end
    local output = "A* path from "..toVectorFormat(startNodeIndex).." to "..toVectorFormat(endNodeIndex)
    .. " distance is "..findNodeByIndex(closeList, endNodeIndex).G.." \n"
    for i = #path, 1, -1 do
        local str = toVectorFormat(path[i].index)
        output = output .. str .. " -> "
    end
    print(string.sub(output, 1, #output - 4))
else
    print("no path found in map.")
end

程序输出（不开启斜墙判断）：

1
2
A* path from (5, 1) to (5, 9) distance is 9.656 
(5, 1) -> (5, 2) -> (5, 3) -> (5, 4) -> (5, 5) -> (6, 6) -> (7, 7) -> (6, 8) -> (5, 9)

路线图：

程序输出（开启斜墙判断）：

1
2
A* path from (5, 1) to (5, 9) distance is 13.07 
(5, 1) -> (5, 2) -> (5, 3) -> (6, 4) -> (7, 4) -> (8, 5) -> (9, 6) -> (9, 7) -> (8, 8) -> (7, 9) -> (6, 9) -> (5, 9)

路线图：

算法
Prev:
鸿蒙cmd
Next:
求最短路径 - Dijkstra算法（lua）

    • Copyright © 2023 oO0OoOo | Powered by Hexo | Theme based Bamboo